- absorption cross section, 3.2
- advanced, 4.0
- Bremstrahlungs, 3.1
- Casimir effect, 2.1
- coherent state, 2.2
- correspondence principle, 2.1
- Coulomb gauge, 2.1
- differential cross section, 4.1
- dipole radiation, 3.1
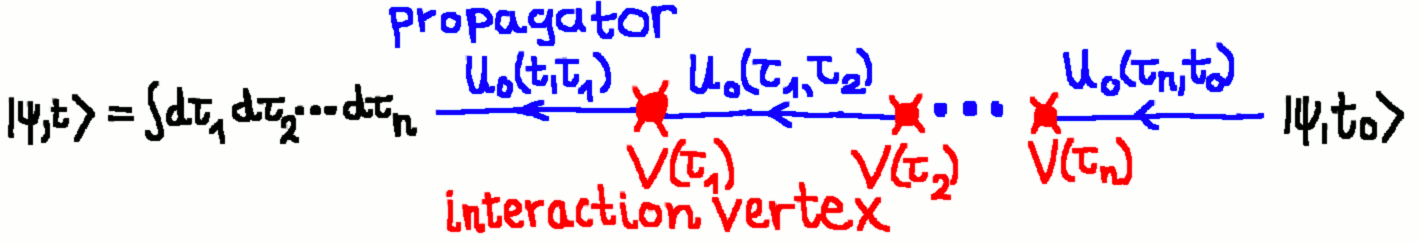
- Dirac picture, 1.1
- Dirac's perturbations theory, 1.1
- far-field approximation, 4.0
- Fermi's golden rule, 1.2
- final state, 1.2
- Glauber state, 2.2
- Greens function, 4.3
|
- Heisenberg picture, 1.1
- homogeneous wave equation, 2.1
- initial state, 1.2
- Lippman-Schwinger, 4.0
- optical theorem, 4.4
- partial-wave amplitude, 4.5
- partial-wave states, 4.5
- photoelectric effect, 3.2
- photon current, 3.2
- photons, 2.1
- Poynting vector, 2.1
- radial Schrödinger equation, 4.5
- resolvent, 4.3
- retarded, 4.0
- s-wave channel, 4.6
- scattering amplitude, 4.0
- scattering phase-shift, 4.5
- spontaneous emission, 3.1
- stimulated emission, 3.1
|
- T-matrix equation, 4.3
- time dependent perturbation theory, 1.1
- time evolution operator, 1.1
- time ordering, 1.1
- transition operator, 4.3
- two-level system, 1.2
- unitarity, 4.5
- wave zone approximation, 3.1
- zero-point energy of the vacuum, 2.1
|